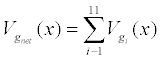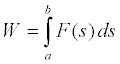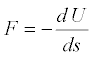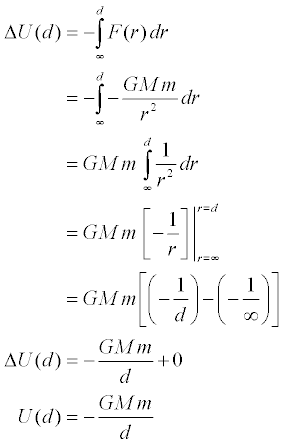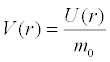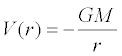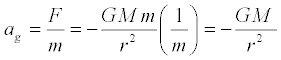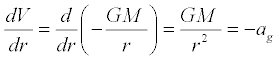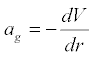The concepts of distance, velocity, acceleration, gravity, mass, and energy are
familiar to anyone who paid attention during Physics 101. As was recently
described in an article in The Physics Teacher, another useful
measure that doesn't usually get too much attention is called potential.1 A
potential is pretty much what it sounds like: a characteristic of something
to potentially do something to something else. Clear, yes? Try this: here,
we present the potential of the gravitational field within the solar system.
In this case, it's the potential of the gravitational field to do work on
mass.
Let's back up and start with work and potential energy.
Work is defined as a force F acting through a distance s.
For a constant force, we have
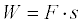
For a variable force, we have
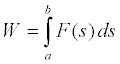
Potential energy is the energy of configuration of a system. We define the
change in potential energy to be the opposite of the work done by a
conservative force--gravity, for example, as opposed to a non-conservative
force like friction--on the components of a system during some change in the
configuration of the system. As an apple falls to the ground, gravity does
work on the Earth-apple system (it exerts a force through a distance),
bringing their centers of mass closer, and in so doing reduces the potential
energy of the Earth-apple system.
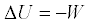
and therefore

which also means that
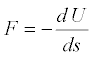
Note that potential energy properly applies to a system, not to the
individual components of the system. With that in mind, it is also very
common to refer to the potential energy of an individual, relatively small
component of a system when there exists a relatively large component. In the
Earth-apple system, the force of gravity acts symmetrically on the Earth and
the apple, producing a force on each of roughly one Newton. While one Newton
is more than enough force to noticeably accelerate an apple, it doesn't accelerate
the Earth very much at all because Earth's mass is so large. We can
therefore speak of the potential energy of a small component of the system
with the understanding that while such use is not completely correct, it is
correct enough.
One nice thing about potential energy is that we can define the
configuration of the system for which the potential energy is zero as
any configuration we want. We usually pick a configuration that makes a
given analysis easy. For the Earth-apple system, we would pick the
configuration of the apply lying on the ground as the zero potential energy
configuration. We would then say (mindful of the caveat in the paragraph
above) that as the apple hangs on the tree, it has some positive amount of
gravitational potential energy. As the apple falls, gravity does work on the
system, the change in potential energy is negative, and the apple's
potential energy is reduced to zero.
Sometimes it's not convenient to use such a configuration to define zero
potential energy. In astronomical work, for example, the potential energy of
a system is defined to be zero when the components are an infinite distance
apart. That may seem strange at first, but it has the advantage of giving
everything in the universe equal footing.
Regardless of the initial configuration used, the potential energy of a
system is equal to the work done by an external force in assembling the
system starting from the initial configuration.
For example, let's calculate the gravitational potential energy of the
Earth-Moon system. We claim that at infinite separation, the potential
energy of the Earth-Moon system is zero. We then find the work done to
bring the Earth and Moon together. Once we have that, we have the
potential energy of the system.
If we bring the Earth and Moon together from infinite separation to distance d, we have
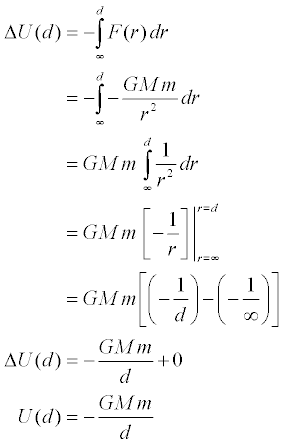
The last two steps show why it is common practice to set U(∞)
= 0 when great distances are involved—because
the potential energy would "naturally" be zero at an infinite separation.
Now all we need are the constants:
|
|
|
|
| G |
= |
6.6743×10-11 m3·kg-1·s-2 |
Universal gravitational constant2 |
| M |
= |
5.9736×1024 kg |
Mass of the Earth |
| m |
= |
7.3490×1022 kg |
Mass of the Moon |
| d |
= |
3.844×108 m |
Distance between Earth and Moon |
We obtain -7.62×1028 Joules. In other
words, to move the Moon away from Earth to an infinite distance through
the force of their mutual gravitational attraction, some outside agent would
have to insert 7.62×1028 Joules into the Earth-Moon system.
This is equivalent to about three minutes and eighteen seconds worth of the
Sun's total energy output, or roughly 150 million years worth of the energy consumed by the
human population, assuming the global consumption of energy were to remain constant
at its 2005 level. 3,4
Potential at a given location is the potential energy
per unit mass, sort of a "specific potential energy" if you will.
Potential is defined as follows
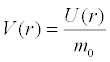
where m0 is a unit mass. With this definition, we see that the potential of the
gravitational field around a point mass is
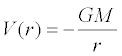
Since the acceleration due to gravity is described by
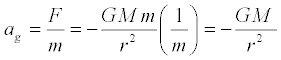
then, in the same manner that force is related to potential energy,
acceleration is related to potential by
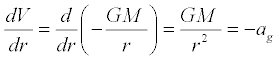
and therefore
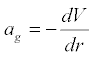
To describe the gravitational potential of the solar system, we simply add
the potentials of the Sun and the planets, taking into account the distance
of each from the Sun. We define a gravitational potential function for each
astronomical body under two conditions: locations outside of the
astronomical bodies and locations within those bodies. Here, we create a
piecewise function such that the gravitational potential within the bodies
is equal to that at their surfaces. This is not entirely meaningful, but it
does make the function continuous.

where
|
|
|
|
| Vgnet(x) |
= |
Net gravitational potential, J |
| G |
= |
6.6743×10-11 m3·kg-1·s-2 |
Universal gravitational constant 2 |
| Mi |
= |
Mass of the ith body, kg |
| Ri |
= |
Semi-major axis of the orbit of the ith body, m |
| ri |
= |
Radius of the ith body, m |
| x |
= |
Radial distance from center of the Sun,
m |
We then sum the potentials to find the net gravitational potential.
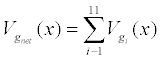
where the constants for the selected bodies have the values described in
the table below.4
|
| i |
Body |
Mass Mi (kg) |
Radius ri (m) |
Semi-major axis Ri (m) |
| 1 |
Sun |
1.9891×1030 |
6.6960×108 |
0 |
| 2 |
Mercury |
3.3020×1023 |
2.4397×106 |
5.7910×1010 |
| 3 |
Venus |
4.8685×1024 |
6.0518×106 |
1.0821×1011 |
| 4 |
Earth |
5.9736×1024 |
6.3710×106 |
1.4960×1011 |
| 5 |
Moon |
7.3490×1022 |
1.7371×106 |
1.4998×1011 |
| 6 |
Mars |
6.4185×1023 |
3.3895×106 |
2.2792×1011 |
| 7 |
Jupiter |
1.8986×1027 |
6.9911×107 |
7.7857×1011 |
| 8 |
Saturn |
5.6846×1026 |
5.8232×107 |
1.4335×1012 |
| 9 |
Uranus |
8.6832×1025 |
2.5362×107 |
2.8725×1012 |
| 10 |
Neptune |
1.0243×1026 |
2.4622×107 |
4.4951×1012 |
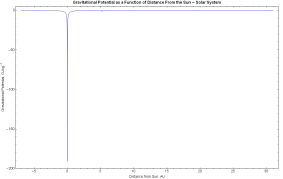
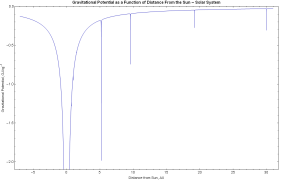
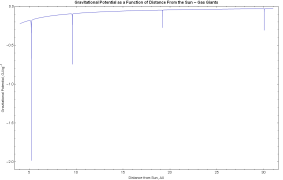
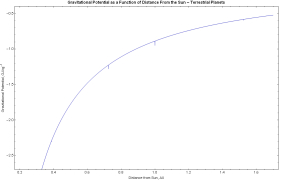
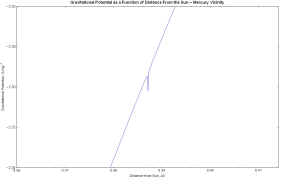
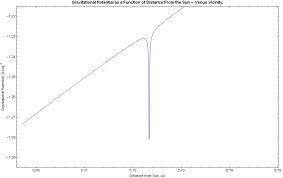
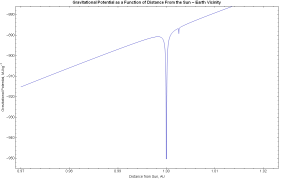
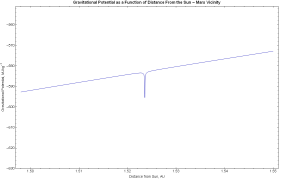
Plots of the gravitational potential function over several regions of the
solar system were made using Mathematica. The plots assume that all astronomical bodies
lie in the plane of the ecliptic and are in conjunction on the same side of
the Sun; i.e., they lie along a straight line
in space with the sun located at r = 0. The values in the domain r < 0 represent the potential
on the side of the Sun opposite that of the
planets. The plot domains are scaled in Astronomical Units where 1
AU = 1.49598×1011 meters.5
Click on the plot thumbnail for a high resolution image
best viewed with a 1280x1024 screen resolution. The Mathematica notebook source code is available here.
The whole solar system
The gas giants
Mercury through Jupiter

The terrestrial planets
References
1 van den Berg, Willem, "The Gravitational Landscape of the Solar System", The Physics
Teacher, Sept. 2008, pp. 363-4.
2 NIST - CODATA Value: Newtonian constant of gravitation.
3 Energy
Information Administration - World Primary Energy Consumption (Btu),
1980-2005.
4 NASA/GSFC -
Planetary Fact Sheets.
5 NASA/JPL - AU Definition. |